
Publications 2019
Dogan MF., Williams-Pierce C. (2019) Supporting Teacher Proving Practices with Three Phases of Proof. Teacher Education Advancement Network Journal, University of Cumbria, 11(3), 48-59
Edwards LD. (2019) The body of/in proof: an embodies analysis of mathematical reasoning. Interdisciplinary Perspectives on Math Cognition, 119-139
Ferreira MBC, Almoulou SA. (2019) Proposta metodológica para o ensino de quadriláteros via provas e demonstrações. Horizontes – Revista de Educação, 7(13), 132-156
Laamena CM., Nusantara T. (2019) Prospective mathematics teachers’ argumentation structure when constructing a mathematical proof: The importance of backing. Beta: Jurnal Tadris Matematika, 12 (1), 43-59
Haj-Yahya A. (2019) Do prototypical constructions and self-attributes of presented drawings affect the construction and validation of proofs? Mathematics Education Research Journal, 1-34
Tråi S (2019) Bevis i undervising av realfagsmatematikk i vidaregåande skule. Bergen University
Bourgade JP. (2019) On an axiological dimension of rigour in school mathematics. Educação Matemática Pesquisa: Revista do Programa, 21(4), 171-184
Mithalal J., Balacheff N. (2019) The instrumental deconstruction as a link between drawing and geometrical figure. Educational Studies in mathematics, 100 (2) 161-176.
Boldrini Cabral S.A. (2019) Uma análise do Pensamento Argumentativo Geométrico com Atividades de Provas Experimentais. Revista do Instituto de Ciēncias Humanas, 15 (21), 19-35
Arzarello F., Soldano C. (2019) Approaching proof in the classroom through the logic of inquiry. In Gabriel Kaiser, Norma Presmeg, Compendium for Early Career Researchers in Mathematics Education, ICME-13 Monograph, pp. 221-243
Weinberg P. (2019) Generalizing and Proving in an Elementary Mathematics Teacher Education Program: Moving Beyond Logic. EURASIA Journal of Mathematics, science and technology education, 15(9).
Publications 2018
Bustos Á, Zubieta G. (2018) La validación matemática como proceso de construcción colaborativo. Una experiencia con ACODESA. Acta Latinoamericana de Matemãtica Educativa, 31(2), 1288-1293
Eliseo N. (2018) Ingeniería didáctica del proceso de prueba en estudiantes universitarios. Acta Latinoamericana de Matemãtica Educativa, 31(1), 334-341
Articles in ZDM, Vol. 51, Issue 5 (2019) - Mathematical evidence and argument
Sommerhoff D., Ufer S. (2019) Acceptance criteria for validating mathematical proofs used by school students, university students, and mathematicians in the context of teaching. ZDM 51 (5) 717-730
Kempen L., Biehler R. (2019) Fostering first-year pre-service teachers’ proof competencies. ZDM 51 (5),731-746
Brunner E., Reusser K. (2019) Type of mathematical proof: personal preference or adaptive teaching behavior? ZDM 51 (5), 747-758
Mariotti M.A, Pedemonte B. (2019) Intuition and proof in the solution of conjecturing problems. ZDM 51 (5), 759-777
Baccaglini-Frank A. (2019) Dragging, instrumented abduction and evidence, in processes of conjecture generation in a dynamic geometry environment. ZDM 51 (5), 779-791
Antonini S. (2019) Intuitive acceptance of proof by contradiction. ZDM 51 (5), 793-806
Reid D.A., Vallejo Vargas E.A. (2019) Evidence and argument in a proof based teaching theory. ZDM 51 (5), 807-823
Aberdein A. (2019) Evidence, proofs, and derivations. ZDM 51 (5), 825-834
Sorensen H.K., Danielsen K., Andersen L.E. (2019) Teaching reader engagement as an aspect of proof. ZDM 51 (5), 835-844
Nickel G. (2019) Aspects of freedom in mathematical proof. ZDM 51 (5), 845-856
Rittberg C.J., Kerkhove B.V. (2019) Studying mathematical practices: the dilemma of case studies. ZDM 51 (5), 857-868
Working Group: International Perspective on Proof and Proving – PME 43
July 07-12, 2019 Pretoria, South Africa
David Reid, Keith Jones and Ruhama Even.
The aim of the group was to foster research on proof and proving from an international perspective, by organising networks of researchers interested in collaborating on comparative research focussed on proof and proving.
Among the participants in the PME WG there was interest in continuing to work on several sub-topics:
- Pre-Primary and Primary Argumentation and Proof
- Secondary Level Argumentation and Proof
- University Level Proof Teaching and Learning
- Proof in the Primary & Secondary School Curriculum
- How are Argumentation and Proof conceptualised internationally?
- Interrelationships between Visualisation and Proving
The short term plan is for interested researchers to conduct research projects on each of these topics in the next year, and to report back (either in person, or in writing) to the PME WG at PME 44. The long term plan is to present the outcomes of these projects in a research forum at PME 45 and/or in an edited book.
Readers of the Newsletter are welcome (encouraged) to join in the research projects. All levels of participation are needed, from being fully involved in planning and conducting the research, to providing information about your country. If you are interested in participating, please contact the coordinators listed below.
Thesis: Assessing ITP Students' Validating Ability: Framing, Developing and Validating a Pilot Assessment
Joshua B. Fagan (2019)
In this paper I discuss the process of creating a closed-form multiple-choice assessment of students’ ability to validate mathematical proofs at the introduction to proof (ITP) level. This process involved:
- creating and validating a cohesive framework of common validity issues (CVI) in proof writing as a basis for assessment creation through a mathematician survey (N = 228) and two focus groups (N = 4 & N = 7);
- creating and piloting an open version of the assessment as a means to create distractors for the closed assessment;
- creating, piloting (N = 187) and analyzing the results from the closed form assessment; and
- conducting interviews with student participants after the pilot to determine the characteristics of the process that students took during the pilot.
The results of the processes offer an assessment that, with some refinement, can authentically measure students’ ability to validate mathematical arguments from a number of perspectives in the ITP setting.
The Genre(s) of Argumentation in School Mathematics
Kristen N. Bieda, David M. Bowers Valentin A.B. Küchle
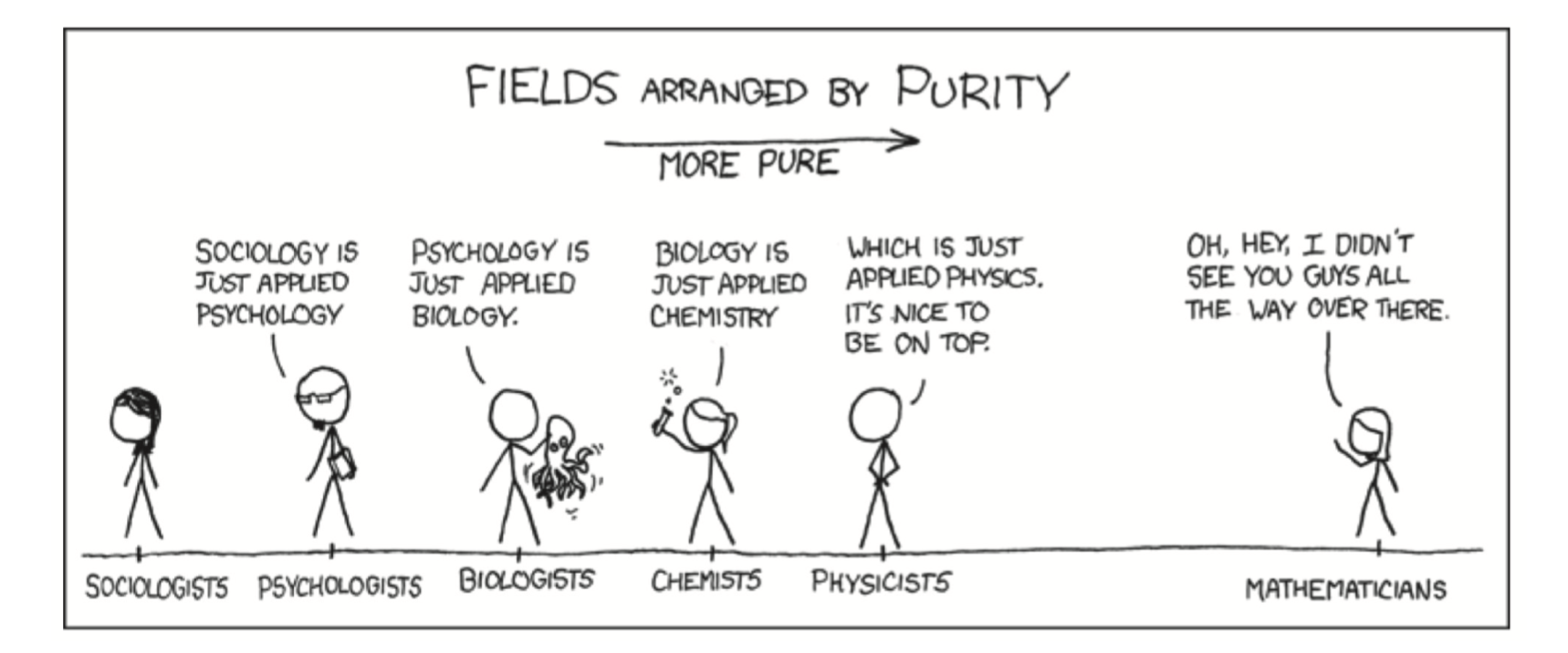
Some see mathematics as a pure and unadulterated expression of our logical and rational faculties. From this commonplace perspective, even heavily mathematical disciplines such as statistics or physics are somehow lesser chimera, beautiful reflections that are nonetheless impure. In truth, this perspective does a very real disservice to mathematics, ignoring as it does the very human and aesthetic considerations of those who practice mathematics (Ernest, 1991; Lakoff & Núñez, 2000). Mathematics, as a body of knowledge, owes much to the art of rigorous logical argument. Rigor and abstraction are manifestations of values held by the community of mathematicians that set norms for how mathematicians communicate with one another as well as how understanding is conceived within the discipline.
Special Day in Honor of Viviane Durand-Guerrier
March 26, 2020 - Bizerte Tunisia
This day is scheduled the day before the INDRUM Conference - Third Conference of the International Network for Didactic Research in University Mathematics (27-29 March 2020).
Editorial Board
Editors-in-chief –
Bettina Pedemonte,
Maria-Alessandra Mariotti
Associate Editors –
Orly Buchbinder,
Kirsti Hemmi,
Mara Martinez
Redactor –
Bettina Pedemonte
Scientific Board –
Nicolas Balacheff,
Paolo Boero,
Daniel Chazan,
Raymond Duval,
Gila Hanna,
Guershon Harel,
Patricio Herbst,
Celia Hoyles,
Erica Melis,
Michael Otte,
Philippe Richard,
Yasuhiro Sekiguchi,
Michael de Villiers,
Virginia Warfield